
Eudoxus of Cnidus was well known for his two major contributions in mathematics: the theory of proportions and his method of exhaustion. Eudoxus was born around 395-390 BC in Cnidus, Asia Minor, which is now known as Turkey. Despite the Eudoxus’s work being lost, many mathematicians throughout history have referred to his work; including, the famous Euclid in his book V Elements dealing with the theory of proportions and book X dealing with the method of exhaustion. Eudoxus’s contributions are fundamental tools for the composition of further important mathematical theories and proofs.
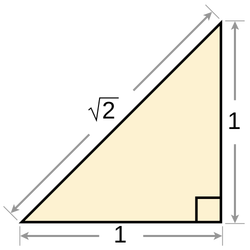
During Eudox’s time period, the Pythagoreans had come up with the idea of commensurability. Commensurability means that the ratio of lengths of two line segments can be express as rational numbers. This idea was later rejected by a counter example in where the ratios could not be express as whole numbers; in other words, the ratios could only be expressed with irrational numbers, as the illustration on the left shows. Thus Eudoxus came up with his theory of proportions, which resolved many of the problems that were doubtfully proven by commensurability. His theory is now found in Book V Euclid’s Elements.
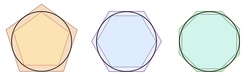
Eudoxu’s second major contribution is the method of exhaustion, now known as a limit. Eudoxus calculated the curvilinear areas and the volumes of regular polygons. With this method, “the successive inscribed figures ‘exhaust’ the original figure” (Wise, David). This technique lasted for 2 millenniums, until calculus was invented in the 17th century. With this technique, the great Archimedes was able to approximate the circumference of a circle.
Both of Eudoxus’ contributions set the stage for Euclid and Archimedes. He is said to be the second greatest mathematician of antiquity, Archimedes being the first. Thanks to Eudoxu’s theory of proportions we have irrational numbers, such as pi and square root of two, and thanks to his method of exhaustion mathematicians were able to approximate the curvilinear areas and volumes of curve shapes and figures for almost 2000 years. Eudoxus and his two major advances in mathematics are worthy of recognition.
Reference
Mendell, Henry Ross. “Eudoxus of Cnidus” Encyclopaedia Britannica. 21 Feb. 2012. Web. 23
Jan. 2014. http://www.britannica.com/EBchecked/topic/195005/Eudoxus-of-Cnidus
Wise, David. “Eudoxus’ Influence on Euclid’s Elements with a close look at The Method of
Exhaustion” The University of Georgia. Web. 23 Jan. 2014 http://jwilson.coe.uga.edu/EMT668/EMAT6680.F99/Wise/essay7/essay7.htm
Krimson. “The Method of Exhaustion is not a method, and exhaustive in a very particular
sence.” Everything2. 2 May, 2002. Web 23. Jan. 2014. http://everything2.com/title/Method+of+Exhaustion
Mendell, Henry Ross. “Eudoxus of Cnidus” Encyclopaedia Britannica. 21 Feb. 2012. Web. 23
Jan. 2014. http://www.britannica.com/EBchecked/topic/195005/Eudoxus-of-Cnidus
Wise, David. “Eudoxus’ Influence on Euclid’s Elements with a close look at The Method of
Exhaustion” The University of Georgia. Web. 23 Jan. 2014 http://jwilson.coe.uga.edu/EMT668/EMAT6680.F99/Wise/essay7/essay7.htm
Krimson. “The Method of Exhaustion is not a method, and exhaustive in a very particular
sence.” Everything2. 2 May, 2002. Web 23. Jan. 2014. http://everything2.com/title/Method+of+Exhaustion
 RSS Feed
RSS Feed